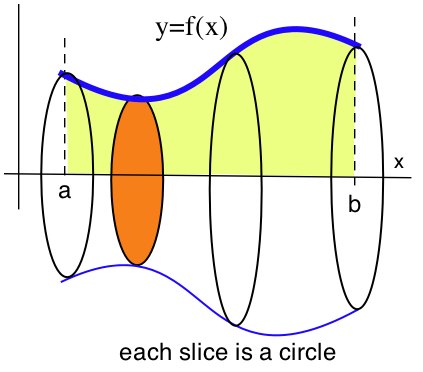
 The region between and the -axis between and is rotated around the -axis to form a solid. Represent the volume of this solid
as a definite integral. Use exact values.
The region between and the -axis between and is rotated around the -axis to form a solid. Represent the volume of this solid
as a definite integral. Use exact values.Volume
 The region between and the -axis between and is rotated around the -axis to form a solid. Represent the volume of this solid
as a definite integral. Use exact values.
The region between and the -axis between and is rotated around the -axis to form a solid. Represent the volume of this solid
as a definite integral. Use exact values.